Quite a few big ideas have made it across from evolutionary theory into the human sciences in the last few years. I can’t think of any that has been more culturally successful than the ‘live fast, die young principle’. This principle, which was originally articulated by George C Williams in the late 1950s, says something like the following: if you live in a world where the unavoidable risk of mortality is high, you should prioritise the present relative to the future. Specifically, you should try to reproduce sooner, even at the expense of your neglected body falling apart down the line. After all, what is the point in investing in longevity when some mishap will probably do you in anyway before you reach a peaceful old age?
The principle was originally invoked to explain inter-species differences in the timing of reproduction, and in senescence (the tendency of bodies to fall apart in multiple ways after a certain number of years of life, without clear external causes). But it has come to crop everywhere: in psychology (to explain individual differences in impulsivity, and the impact of early-life trauma), in sociology (to explain socioeconomic differences) , in anthropology and history (to explain social change). I’ve even found it invoked in explaining how travel agencies responded to the disruption caused by the pandemic. And then of course, there is the famous story of Henry Ford, asking engineers to tour the scrapyards of America, finding parts of model T cars that were still in good condition in scrapped carcasses. They found that the kingpin was never worn out; ‘make them less well!’, came the response.
On the face of it, this is a beautiful example of theory guiding observation, science in the hypothetico-deductive mode working well. Williams produced an a priori theoretical argument. Subsequent data from many species supported its central prediction: species that experience a higher mortality rate in their natural environments mature sooner (and smaller); have larger litters; have shorter inter-birth intervals, and may senesce sooner. Then, it was like the joke about confirmation bias: once you are aware of it, you see it everywhere. For people spotting it (for example in the behaviour of travel agencies), it was nice to link back to the prestige of evolutionary biology and the idea that the pattern was predicted by theory. But there is a problem. The problem is not with the bit that says that the living fast and dying young pattern of behaviour occurs; this does indeed seem to be an empirical regularity of some generality. The problem lies in the bit that says theory predicts it will occur.
It seems to be a well-kept secret that there is no consensus in evolutionary biology that Williams’ theoretical argument was correct. That’s putting it mildly. As a 2019 review in Trends in Ecology and Evolution put it: ‘[Williams’] idea still motivates empirical studies, although formal, mathematical theory shows it is wrong’. The authors of that paper suggest that Williams’ argument persists not because it is sound, but because it is intuitive. Intuitive it definitely is. I remember acting as editor for another important paper showing mathematically that –other things being equal — the risk of unavoidable mortality can have no impact on the optimal timing of reproduction, or any other trade-off parameter for that matter. And I remember thinking: but it obviously must, you need to rewrite the equations until they say so! (I didn’t say this in my editorial review of course).
The difficulty is that, until now, there has been no explanation of why Williams’ argument does not work that is anything like as intuitive as the original argument was. A new paper by Charlotte de Vries, Mattias Galipaud and Hanna Kokko comes as close as anything I have ever encountered to giving me an intuitive magic for the failure of the argument that is nearly strong enough to battle the intuitive magic of the argument itself. (For those of you who don’t know Hanna, the combination of brilliant theoretical insight and limpid clarity in communication is exactly the kind of behaviour she has form for.) The paper, as well as explaining the difficulty with Williams’ original argument, signposts the ways we might rescue the live fast, die young principle, and in the process shows how the scientific method – theory leads to prediction leads to test – is not entirely as we like to imagine.
Alright, let’s roll our sleeves up. Let us imagine a population living in a dangerous world, whose members put everything into reproducing in the first year of their lives. Then they are knackered, and die off even if the dangers of their world haven’t got to them first. There is no selection for reproducing less when young and being healthy for a second year, because so few are going to make it that far anyway. Now, due to a change in the ecology, the rate of unavoidable mortality goes down. Now, many more individuals can be around in the second year. By reproducing a bit less in the first year, individuals can be in better health in the second year, leading to higher total lifetime reproductive success. And this delaying is now more worth doing, because they now have a better chance of making it through and reaping the benefit. This is Williams’ original argument, and it still seems to make a lot of sense.
We are talking about evolution though, as Ernst Mayr taught us, thinking about evolution requires thinking about populations, not about isolated individuals. If the rate of mortality goes down, the population is going to grow exponentially, at a faster rate than it did previously. In an exponentially growing population, an offspring that you have sooner is more valuable in fitness terms than one you have later. De Vries et al. give us a nice figure to see why this is the case.
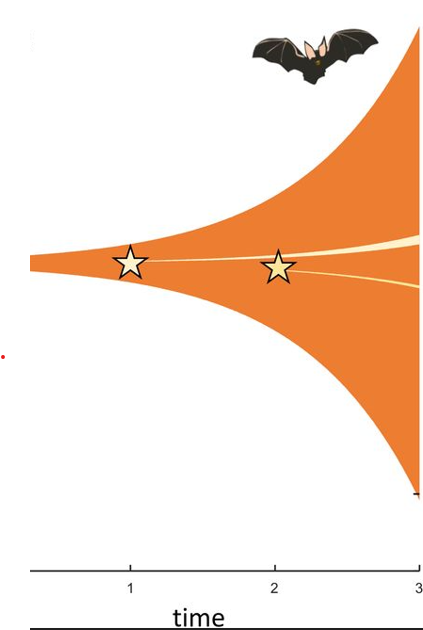
The thing that gets maximised by evolution is the proportionate representation in the population of some lineage or type. And it is easy to see that in a growing population, an offspring placed into the population earlier (the left hand star on the figure) can become ancestor to a greater fraction of the population by time 3 than an offspring placed into the population later (the right hand star). This is because it is placed in when the cone is narrower, and its descendants begin their exponential growth in number sooner. So, when a population is growing exponentially, there is a fitness bonus attaching to any offspring you manage to have soon. To look at this the other way about, there is a relative fitness penalty attached to any offspring you have later in time.
So when the rate of unavoidable mortality goes down, two things happen. The chances of making it to a second year goes up, increasing the expected return on investments in reproductive capacity in the second year. And, because the population begins to grow exponentially, the relative fitness penalty for an offspring being placed a year later gets bigger. Your chances of having an offspring in the second year go up; and the relative value to your fitness of a offspring a year later goes down; and these two effects perfectly cancel one another. The change in the risk of unavoidable mortality ends up having no effect at all on your optimal trade-off between reproduction and health.
Aha, you might say. But this penalty for delayed offspring only applies in exponentially growing populations. So Williams’ argument would work in a population where mortality decreased, but the population size remained stable. Indeed, but the trouble is that the only thing that keeps populations from growing exponentially is mortality. Imagining mortality reducing without that causing the population to grow exponentially is like imagining putting air into a balloon without that balloon starting to get bigger.
Exponentially growing populations are eventually limited and stabilized by competition amongst their members (so-called density-dependent regulation). The way to rescue Williams’ argument is to incorporate some kind of density-dependent regulation that checks population growth, but still rewards those who delay reproductive effort. The problem is that there are many ways that density-dependent regulation can work (as competition increases, the old lose out, the young lose out, fecundity is reduced amongst inexperienced breeders, it is reduced amongst experienced breeders, new juveniles can’t find nest sites, old adults can’t defend their nest sites, etc.). de Vries et al. consider ten different scenarios for density-dependent regulation. They find that under some of these, reducing unavoidable mortality selects for delaying reproduction (the Williams pattern); in some reducing unavoidable mortality selects for accelerating reproduction (the anti-Williams pattern); and in some, reducing unavoidable mortality has no effect either way.
There is perhaps no way of saying a priori which of the ten scenarios for density-dependent regulation actually captures what happens in any particular real population. So, we can’t know a priori what our theoretical model should be. Instead, de Vries et al. say, reasonably enough, we can use the fact that we do observe the live fast, die young pattern across many species to narrow down what the right theoretical model is. In other words, we can predict what our theory should be using data. That is, since populations with higher mortality do evolve earlier reproduction, we can infer that they should be modelled using the models in which this is predicted to happen. Those are models where the burden of density-dependent competition falls particularly on juveniles trying to start reproducing, or on fecundity. So, since we stumbled on empirical regularity (populations with higher mortality evolve earlier reproduction and senescence), we learned something, indirectly, about what assumptions we should make about how populations work.
I take a few lessons away three lessons from this example. One, when people say ‘evolutionary theory predicts…..’, they are often just peddling an intuition, an intuition that may not work out, or may only work out under restrictive assumptions. In this case, if we wanted to say ‘theory predicts’ the live fast die young pattern, what we ought to say ‘perfectly reasonable theory predicts either this pattern, or the opposite, or no pattern at all, depending on assumptions’.
Two, our intuitions don’t do population thinking. We compute what the payoff to one individual would be of doing A rather than B. We don’t spontaneously think about what the changing population distribution would be if A rather than B become common. But evolution is a population process, and so you can’t work out what will happen without modelling populations (you often get misleading results just by totting up costs and benefits to one representative individual).
Third, theory does not really come before data, even in a relatively ‘theoretical’ and mathematically formalized discipline like evolutionary biology. It’s the empirical findings that tell us what kinds of theoretical models we should construct, and how to constrain them. This is worth noting for those who are advocating more formal theory as a way out of psychology’ current crisis. Sure, we need to build more models, but model-making prior to any data is blind. You don’t just figure out the right model in a vacuum and go off and test it. Data – observation of the world, often rather descriptive and interest-driven – tell us what theoretical assumptions we should be making, almost as much as theoretical models then tell us which further data to collect. It’s a cycle, a game of tag between models and data, in which data predict theory as well as the opposite.
Subscribe to this blog by entering your email in the subscribe box on the right. Regular posts on psychology, behavioural science and society.